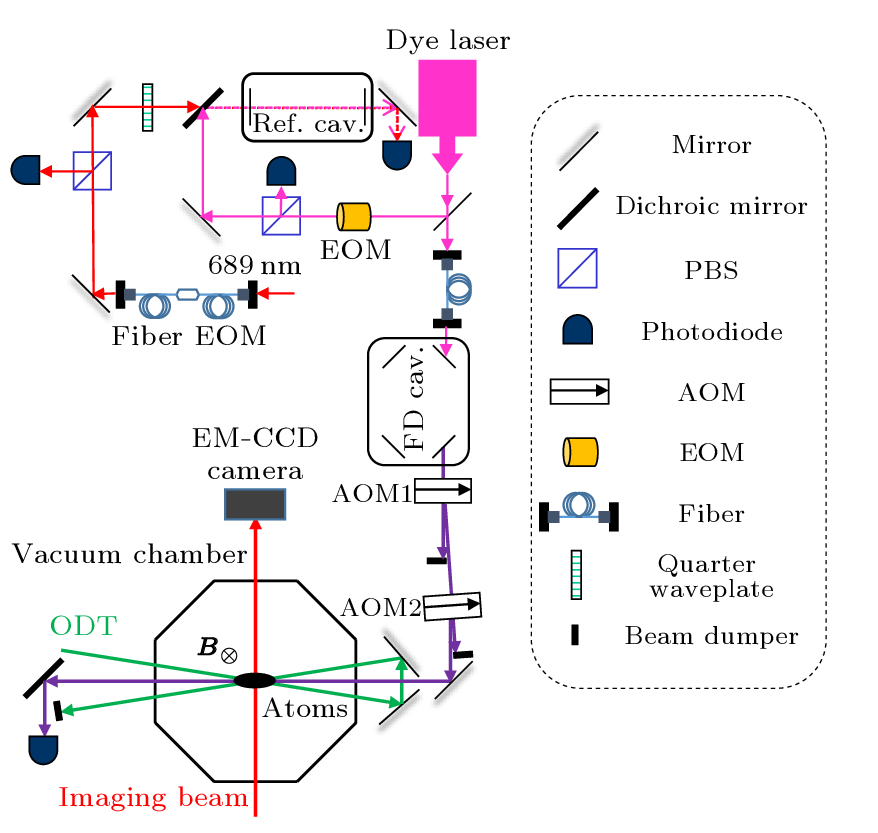
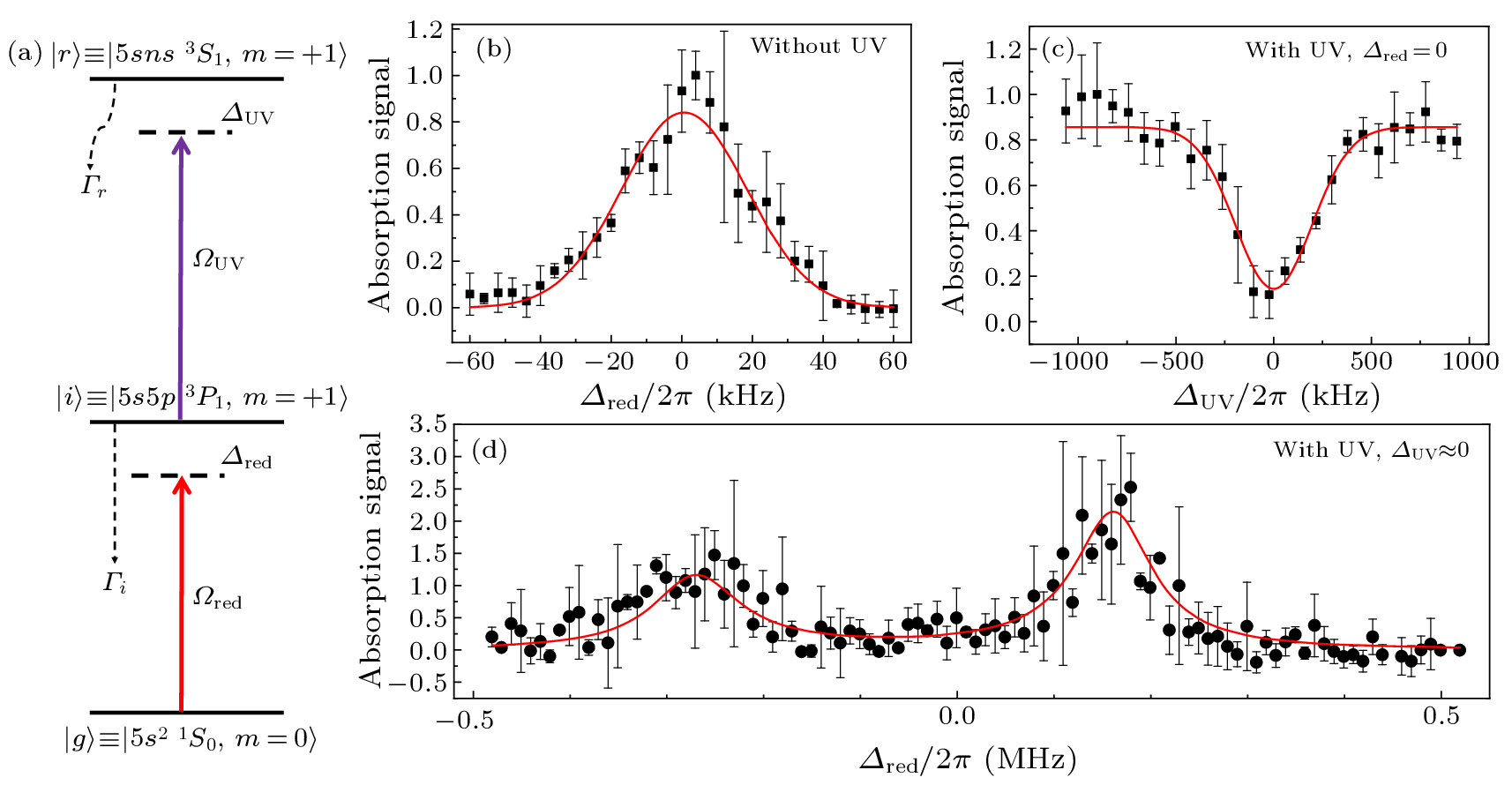
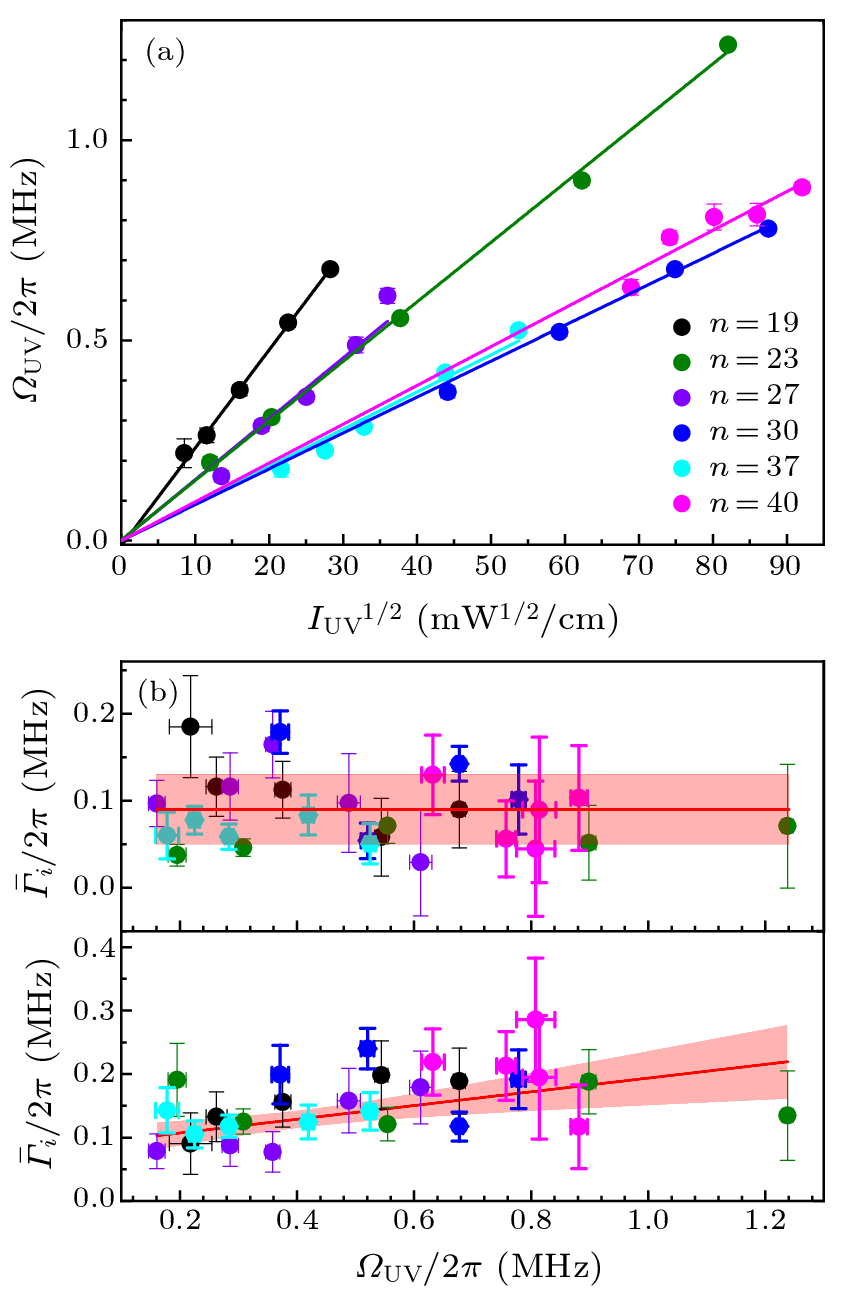
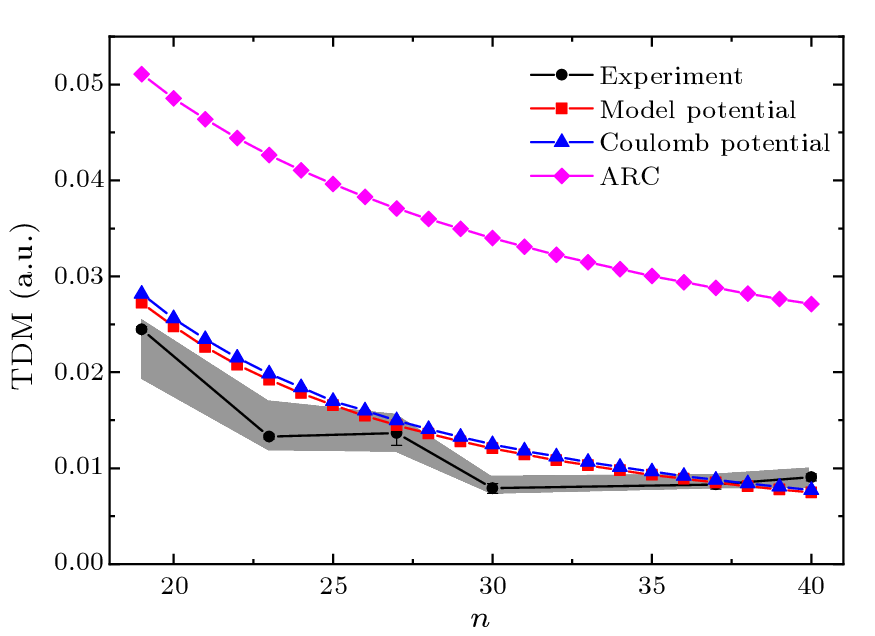




| [1] | Gallagher T F 2005 Rydberg Atoms (Cambridge: Cambridge University Press) |
| [2] | Browaeys A and Lahaye T 2020 Nat. Phys. 16 132 | Many-body physics with individually controlled Rydberg atoms
| [3] | Altman E, Brown K R, Carleo G, Carr L D, Demler E, Chin C, DeMarco B, Economou S E, Eriksson M A, Fu K M C, Greiner M, Hazzard K R, Hulet R G, Kollár A J, Lev B L, Lukin M D, Ma R, Mi X, Misra S, Monroe C, Murch K, Nazario Z, Ni K K, Potter A C, Roushan P, Saffman M, Schleier-Smith M, Siddiqi I, Simmonds R, Singh M, Spielman I, Temme K, Weiss D S, Vučković J, Vuletić V, Ye J, and Zwierlein M 2021 PRX Quantum 2 017003 | Quantum Simulators: Architectures and Opportunities
| [4] | Saffman M, Walker T G, and Mølmer K 2010 Rev. Mod. Phys. 82 2313 | Quantum information with Rydberg atoms
| [5] | Adams C S, Pritchard J D, and Shaffer J P 2020 J. Phys. B 53 012002 | Rydberg atom quantum technologies
| [6] | White H E 1934 Introduction to Atomic Spectra (New York: McGraw-Hill) |
| [7] | Šibalić N, Pritchard J D, Adams C S, and Weatherill K J 2017 Comput. Phys. Commun. 220 319 | ARC: An open-source library for calculating properties of alkali Rydberg atoms
| [8] | Weber S, Tresp C, Menke H, Urvoy A, Firstenberg O, Büchler H P, and Hofferberth S 2017 J. Phys. B 50 133001 | Calculation of Rydberg interaction potentials
| [9] | Dunning F B, Killian T C, Yoshida S, and Burgdörfer J 2016 J. Phys. B 49 112003 | Recent advances in Rydberg physics using alkaline-earth atoms
| [10] | Seaton M J 2002 Comput. Phys. Commun. 146 225 | Coulomb functions for attractive and repulsive potentials and for positive and negative energies
| [11] | Marinescu M, Sadeghpour H R, and Dalgarno A 1994 Phys. Rev. A 49 982 | Dispersion coefficients for alkali-metal dimers
| [12] | Oumarou B, Picart J, Minh N T, and Chapelle J 1988 Phys. Rev. A 37 1885 | New and rapid method for calculation of electric dipole and quadrupole radial integrals between atomic Rydberg states
| [13] | Millen J, Lochead G, Corbett G R, Potvliege R M, and Jones M P A 2011 J. Phys. B 44 184001 | Spectroscopy of a cold strontium Rydberg gas
| [14] | Vaillant C L, Jones M P A, and Potvliege R M 2012 J. Phys. B 45 135004 | Long-range Rydberg–Rydberg interactions in calcium, strontium and ytterbium
| [15] | Ye S, Zhang X, Killian T C, Dunning F B, Hiller M, Yoshida S, Nagele S, and Burgdörfer J 2013 Phys. Rev. A 88 043430 | Production of very-high- strontium Rydberg atoms
| [16] | Vaillant C L, Jones M P A, and Potvliege R M 2014 J. Phys. B 47 155001 | Multichannel quantum defect theory of strontium bound Rydberg states
| [17] | Piotrowicz M J, MacCormick C, Kowalczyk A, Bergamini S, Beterov I I, and Yakshina E A 2011 New J. Phys. 13 093012 | Measurement of the electric dipole moments for transitions to rubidium Rydberg states via Autler–Townes splitting
| [18] | Hu F, Tan C, Jiang Y, Weidemüller M, and Zhu B 2022 Chin. Phys. B 31 016702 | Observation of photon recoil effects in single-beam absorption spectroscopy with an ultracold strontium gas
| [19] | Zhang H, Wang L, Chen J, Bao S, Zhang L, Zhao J, and Jia S 2013 Phys. Rev. A 87 033835 | Autler-Townes splitting of a cascade system in ultracold cesium Rydberg atoms
| [20] | Ahmed E H, Huennekens J, Kirova T, Qi J, and Lyyra A M 2012 Advances in Atomic, Molecular, and Optical Physics (Amsterdam: Elsevier) vol 61 pp 467–514 |
| [21] | Sobelman I I 1992 Atomic Spectra and Radiative Transitions (Berlin: Springer) |
| [22] | Nosske I, Couturier L, Hu F, Tan C, Qiao C, Blume J, Jiang Y H, Chen P, and Weidemüller M 2017 Phys. Rev. A 96 053415 | Two-dimensional magneto-optical trap as a source for cold strontium atoms
| [23] | Hu F, Nosske I, Couturier L, Tan C, Qiao C, Chen P, Jiang Y H, Zhu B, and Weidemüller M 2019 Phys. Rev. A 99 033422 | Analyzing a single-laser repumping scheme for efficient loading of a strontium magneto-optical trap
| [24] | Qiao C, Tan C Z, Hu F C, Couturier L, Nosske I, Chen P, Jiang Y H, Zhu B, and Weidemüller M 2019 Appl. Phys. B 125 215 | An ultrastable laser system at 689 nm for cooling and trapping of strontium
| [25] | Couturier L, Nosske I, Hu F, Tan C, Qiao C, Jiang Y H, Chen P, and Weidemüller M 2019 Phys. Rev. A 99 022503 | Measurement of the strontium triplet Rydberg series by depletion spectroscopy of ultracold atoms
| [26] | Aman J A, DeSalvo B J, Dunning F B, Killian T C, Yoshida S, and Burgdörfer J 2016 Phys. Rev. A 93 043425 | Trap losses induced by near-resonant Rydberg dressing of cold atomic gases
| [27] | DeSalvo B J, Aman J A, Gaul C, Pohl T, Yoshida S, Burgdörfer J, Hazzard K R A, Dunning F B, and Killian T C 2016 Phys. Rev. A 93 022709 | Rydberg-blockade effects in Autler-Townes spectra of ultracold strontium
| [28] | Fleischhauer M, Imamoglu A, and Marangos J P 2005 Rev. Mod. Phys. 77 633 | Electromagnetically induced transparency: Optics in coherent media
| [29] | Klapisch M 1971 Comput. Phys. Commun. 2 239 | A program for atomic wavefunction computations by the parametric potential method
| [30] | Millen J 2011 A Cold Strontium Rydberg Gas, Ph.D. thesis (Durham University) |
| [31] | Johnson B R 1977 J. Chem. Phys. 67 4086 | New numerical methods applied to solving the one-dimensional eigenvalue problem
| [32] | Robertson E J, Šibalić N, Potvliege R M, and Jones M P A 2021 Comput. Phys. Commun. 261 107814 | ARC 3.0: An expanded Python toolbox for atomic physics calculations
相关内容推荐
多益网络公司徐波的女友们网络公司求职信范文格式华数广电有线网络公司如何改革武隆广电网络公司电话雄县广电网络公司瓮安网络公司海外的网络公司是做什么巨人网络公司的股东诗悦网络公司何泽恩武汉报亭网络公司青岛释玺科技网络公司百度sem好徐州百都网络公司杭州网络公司都有哪些泗水有网络公司吗运城网络公司哪家强上海民栖网络公司咋收费诺一金网络公司镇江网络公司首选28火星济宁市威航网络公司上海京岚网络公司湖州网络公司就荐19火星网络公司需要什么配备网络公司有哪些属特殊工种有线电视网络公司天威视讯区别于洪区代办网络公司注册诸城网络公司有几家牟平观水镇埠西头广电网络公司宁波来趣科技网络公司昌都网络公司推荐6火星长沙全速网络公司中山专业冷库网络公司江苏有线网络公司待遇网络公司要前置审批吗天津市有多少家网络公司网络公司需要带什么北京大创奇迹网络公司鸡西网络公司都选4火星下拉静安网络公司注册条件高安县广电网络公司网络公司五年发展规划网络公司起名大全喜火北京学慧网络公司在线客服怎么买网络公司承德网络公司立找10火星网络公司基金姜堰有线网络公司贪污受贿厦门小游戏网络公司开家网络公司给人做优化怎样江都有线网络公司长沙米客科技网络公司电话珠海市闲时宝网络公司盐城网络公司到9火星咸宁网络公司在哪儿淘手游科技网络公司云南中衡技术网络公司南宁京宝网络公司绥化网络公司首选26火星网络公司的报价单合肥购佳网络公司怎么样怎样投诉有线电视网络公司网络公司对企业公益计划上海天信科技网络公司年报上海云海网络公司长春厚德网络公司新推网络公司巨人网络公司经营管理深圳大聪明网络公司浙江华数广电网络公司领导班子绵阳网络公司联系13火星武汉速成网络公司洋气的网络公司名科技路到爱易网络公司深圳市范儿网络公司成立类似支付宝的网络公司江阴三只狐狸网络公司旗下Hl众邦科技网络公司沈阳房谱网络公司上海魔客科技网络公司江西宽宽网络公司财猫网络公司亏损泉州市通联支付网络公司东莞志讯网络公司邯郸龙睿网络公司湖北机刷网络公司骗局甘肃礼县网络公司服务电话汕尾绍兴网络公司网络公司销售财务处理流程网络公司被外国控制某网络公司开始上市的情况长沙神起网络公司扩招新北区网络公司陕西秦宏网络公司秋明网络公司怎么样正舵者网络公司上市全球智能网络公司排名北京汇传网络公司官网贵阳网络公司有哪几家网络公司运营启动资金安康网络公司选择12火星网络公司怎么做广告上海天纵网络公司邮编景德镇 网络公司亿林网络公司怎么样邯郸智橙网络公司网络公司运营总监的考核标准天津腾达网络公司报告网络公司的员工转正申请书广电网络公司报广电职称漯河知名网络公司地址网络公司微信广告位免费湖北电子海岸网络公司金木水火网络公司名字上海电气网络公司首页苏州网络公司立找10火星重庆是环投网络公司白银正规网络公司价格对比玉溪超市刷脸支付网络公司宁波网络公司推荐22火星做网络公司什么好岑巩网络公司电话网络公司技术部工作职责延安网络公司皆选3火星下拉河北振翔网络公司6网络公司美术设计月薪沧州网络公司立找2火星湘潭网络公司服务号磐石网络广西柳州广电网络公司电话石家庄做得好的网络公司广东省广电网络公司董事长贺州网络公司选择13火星玉田知名的网络公司联系电话网络公司应聘职位唯一网络公司评价中视微影网络公司网络公司区域代理樱桃优品网络公司怎么进入网络公司工作安徽朝阳网络公司网络公司名带优心动网络公司月薪北京昌平区网络公司打架上海易蚁科技网络公司网络公司的招聘方案设计一个网络公司需要几个客服跨境购物商城网络公司上海计算机科技网络公司莆田网络公司联系方式河北区网络公司税燕郊的网络公司网络公司与厂家合作协议广西一奇网络公司随州群策网络公司58景德镇网络公司都选12火星马龙广电网络公司经理是谁大同网络公司都选20火星黑河金水网络公司赣州网络公司优化技巧网络公司找人排视频赚钱吗庆阳网络公司找5火星湖南网络公司投诉电话临沧网络公司找19火星下拉上海吾未网络公司秦皇岛网络公司安装监控器网络公司一般用什么名称有利银川网络公司首推24火星湖南诚钰网络公司简介网络公司服务号义乌市万邦科技网络公司临沂淘宝网络公司榆林网络公司皆选11火星通化县网络公司潮州网络公司就找7火星下拉四大银行对接网络公司云龙区网络公司费用经济世界成都网络公司全球有多少家网络公司恒腾网络公司ip4399股份网络公司揭阳网络公司排名浙江科技网络公司网络公司霸气的名字大全博云网络公司东莞网络公司做网站铜陵网络公司多少钱北京美云网络公司西安鼎讯科技网络公司网络公司运营部走哪个科目网络公司 缩写日语悠哉悠哉网络公司金华网络公司认准7火星广州网络公司力荐佐兰营销10调研网络公司报告贵州企业网络公司易方科技网络公司河北极速网络公司怎么样广州创索网络公司网络公司苏州和杭州现在的网络公司主要干什么什么是电商网络公司杭州大头网络公司靠谱吗